Period
2021.06-2022.06
Introduction
Wireless community has made much effort in RFID localization systems, which offer an appealing alternative as it can achieve high-precision, auto-identification, and working with Non-Line-of-Sight (NLoS) cases.
RFID-localization can be broadly classified into two categories: fingerprint and trigonometry. The fingerprint-based methods are built based on the assumption that each distinct location has its own unique RF-signature, while the trigonometry-based methods are generally more accurate as both triangulation and trilateration are derived from fine-grained phase
measurements.
In this project, we present GLAC, the first 3D localization system that enables millimeter-level object manipulation for robotics using only COTS devices. Compared to prior arts, it achieves the most accurate localization while consuming minimal resources in terms of cost-effectiveness and time-efficiency.
Methodology
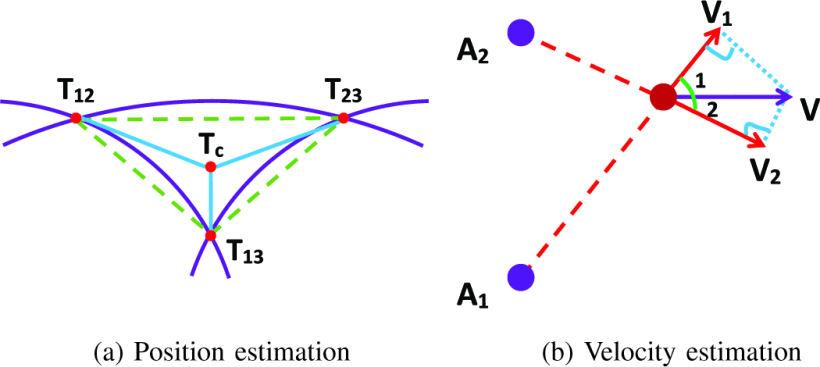
The basic RFID localization problem is that given a time series of phase measurements, finding the most probable location series. We translate this process into an HMM.
To find the optimal trajectory, the simplest way is to compute the likelihood of the observation sequence for each trajectory. Then we choose the trajectory with the maximum observation likelihood among all possibilities. To do so, we need to estimate all the hidden states and this is where Kalman filters come in.
There are several distinct features of our HMM framework with multiple Kalman filters worth mentioning:
- To estimate the next state, we only need a single-phase measurement, which accommodates the asynchronous reading mode and differs from all previous approaches.
- As the reading time is already minimized by using only single-phase updates, we design two techniques to achieve computation efficiency, nearest neighbor pruning and initial state pruning.
Achievement
We evaluate GLAC’s 3D tracking accuracy in both LoS and NLoS scenarios. Figure 6 shows CDFs of position errors for both LoS and NLoS cases. We observe that the median accuracies of all x/y/z dimensions are within one centimeter for all scenarios. In particular, the median accuracies of x/y/z dimensions are 0.35 cm, 0.35 cm and 0.52 cm in LoS settings, while counterparts are 0.57 cm, 0.40 cm and 0.73 cm in NLoS settings, which degrade slightly due to lower SNRs.
